How to find the square of a binomial
The rule to solve the square of a binomial (A + B)2 is the following: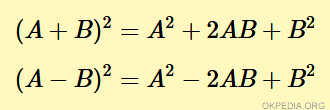 
The square of the binomial (A + B)2 is the sum of
- the square of the first element A2
- double the product of the two terms 2AB
- the square of the second element B2
Examples
Example 1
$$ ( 4 + 2 )^2 $$
$$ ( 4^2 + 2 \cdot 4 \cdot 2 + 2^2 ) $$
$$ ( 16 + 16 + 4 ) = 36 $$
Example 2
$$ ( x - 3 )^2 $$
$$ ( x^2 - 2 \cdot 3x + 3^2 )$$
$$ ( x^2 - 6x + 9 )$$
Example 3
$$ ( 2x + 3y )^2 $$
$$ ( (2x)^2 + 2(2x \cdot 3y) + (3y)^2 ) $$
$$ ( 4x^2 + 12xy + 9y^2 ) $$
https://how.okpedia.org/en/mathematics/how-to-find-the-square-of-a-binomial
Report an error or share a suggestion to enhance this page
